nLab Cardy condition

Idea

The Cardy condition is part of the sewing constraint consistency condition on data that gives an open-closed 2d CFT or 2d TQFT.

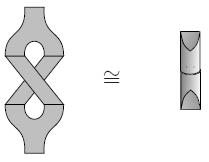
It is the algebraic reflection of the fact that the following two cobordisms are equivalent (as topological as well as as conformal cobordisms):

1. an open string coming in, closing to a closed string and then opening up again to an open string (the “zip-unzip cobordism”);

2. an open string coming in, splitting into two open strings, these crossing each other (with the endpoints of one of them at the same time making a full rotation), then both merging again to one open string.

(e.g. Lauda-Pfeiffer 05 (3.44), Kong 06, figure 3)

For instance in the classification of open-closed 2d TQFT with coefficients in Vect via Frobenius algebras, this means that for $O$ the Frobenius algebra of open string states and for $C$ the commutative Frobenius algebra of closed string states, then the canonical linear function

$$O \longrightarrow C \longrightarrow O$$

is equal to the canonical map

$$O \stackrel{\Delta}{\longrightarrow} O \otimes O \stackrel{\tau}{\longrightarrow} O \otimes O \stackrel{\mu}{\longrightarrow} O \,,$$

where $\mu$ is the coproduct, $\mu$ the product and $\tau$ the braiding (e.g. Lauda-Pfeiffer 05 (2.14)).

Related concepts

• modular invariance

References

Discussion in 2d TQFT includes

• Aaron Lauda, Hendryk Pfeiffer (2005), Open-closed strings: two-dimensional extended TQFTs and Frobenius algebras, Topology Appl. 155, 623-666. (arXiv:0510664)

• Gregory W. Moore, Graeme Segal (2006), D-branes and K-theory in 2D topological field theory. (arXiv:hep-th/0609042)

• Gregory W. Moore, Graeme Segal (2002), Lectures on Branes, K-theory and RR Charges. Lecture notes from the Clay Institute School on Geometry and String Theory held at the Isaac Newton Institute, Cambridge, UK. Available here; related notes from the ITP Miniprogram ‘The Duality Workshop’ at Santa Barbara available here.

Discussion in 2d CFT includes

• Liang Kong, Cardy condition for open-closed field algebras (arXiv:math/0612255)